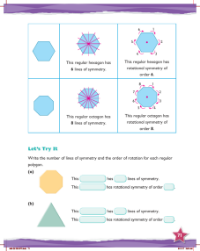
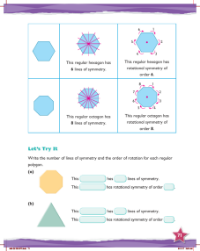
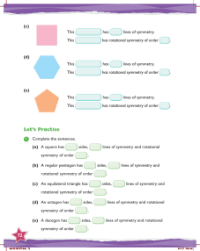
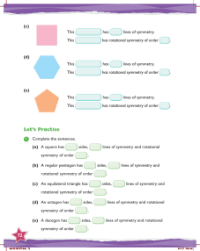
Max Maths, Year 5, Practice, Reflective and rotational symmetry in regular polygons (2)

In a Max Maths activity designed for Year 5 students, the concept of symmetry is explored through the lens of regular polygons. The exercise begins with a true or false section where students must identify accurate statements about the properties of regular polygons. They are presented with three statements: one equating the number of sides to the order of rotational symmetry, another equating the number of sides to the number of lines of symmetry, and the last stating that the number of lines of symmetry equals the order of rotation. Students are encouraged to tick the sentences that correctly describe the properties of regular polygons.

The task then moves on to a hands-on activity using polygon cards. Students are instructed to find and categorize triangles based on their lines of symmetry, noting that triangles can have no lines of symmetry, one line, or three lines, but never two. This is followed by an investigation of quadrilaterals, sorting them according to whether they have no symmetry, one line, two lines, or four lines of symmetry, and discussing why it's impossible for a quadrilateral to have three lines of symmetry. The final part of the activity involves sorting polygons into regular and irregular sets and examining the lines of symmetry and order of rotation for each. This comparison aims to help students observe and understand the differences in symmetry and rotational order between regular and irregular polygons.