Equations with 2 unknown variables

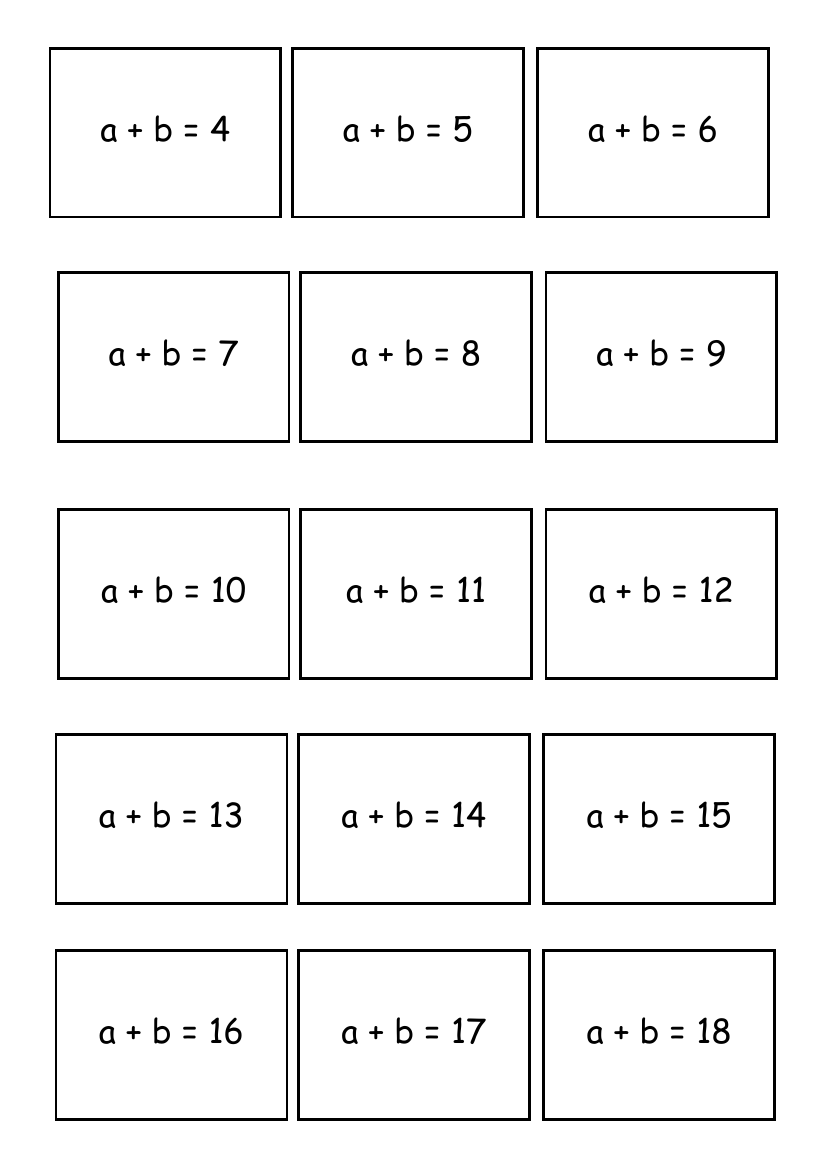
When we look at equations with two unknown variables, such as 'a' and 'b', we are dealing with a system that requires two distinct equations to find unique solutions for each variable. The equations provided here are in two sets. The first set includes equations where the sum of 'a' and 'b' is given, with values ranging sequentially from 4 to 18. Each equation in this set can be represented as a + b = n, where 'n' is the sum, and it takes on values from 4 up to 18 in increments of 1. These equations alone are not sufficient to determine the exact values of 'a' and 'b', as there are infinite combinations of 'a' and 'b' that can satisfy each equation.

The second set of equations provided follows a similar pattern, but instead of the sum, it gives the difference between 'a' and 'b'. Each equation in this second set can be represented as a - b = m, where 'm' is the difference, and it ranges from 4 to 18, also in increments of 1. Like the first set, these equations by themselves do not provide enough information to uniquely determine 'a' and 'b'. However, if we were to pair one equation from the first set with one from the second set, we would have a system of two equations with two unknowns, which can be solved simultaneously to find specific values for 'a' and 'b'. This is a fundamental concept in algebra, where the intersection of solutions from both equations would give us the exact values of the two unknown variables.