Decimal - Vocabulary

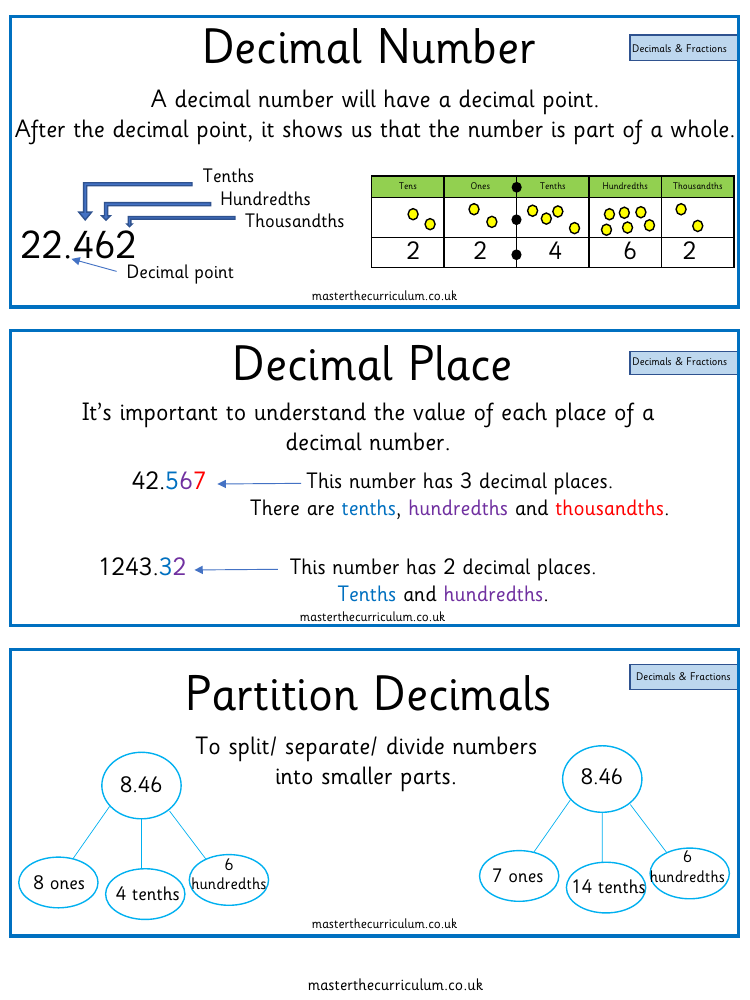
Understanding decimals is a vital part of mathematics, particularly when relating them to fractions. A decimal number is identified by the presence of a decimal point, which indicates that the number is a part of a whole, rather than a whole number itself. Each position to the right of the decimal point represents a specific place value, such as tenths, hundredths, and thousandths. For instance, in the number 22.462, there are tenths (4), hundredths (6), and thousandths (2), amounting to three decimal places in total. In contrast, the number 1243.32 has two decimal places: tenths (3) and hundredths (2). Partitioning decimals involves breaking down these numbers into their constituent parts to better understand their value.

The concept of place value extends to fractions as well. For example, one tenth is represented as 0.1, one hundredth as 0.01, and one thousandth as 0.001. Converting between decimals and fractions is a process of changing the form while keeping the value the same. Simplifying fractions means reducing them to their simplest form without changing their value; for example, 4/8 simplifies to 1/2. When dealing with fractions, the denominator indicates the number of equal parts the whole is divided into, while the numerator represents the number of those parts being considered. Exchanging values within the same place value system, such as converting 10 hundredths (0.1) into 1 tenth, is another way to manipulate these numbers while maintaining their value. Integers, on the other hand, are whole numbers and can be both positive and negative, such as 4, 78, 124, or -34.