Solve problems involving the calculation and conversion of units of measure 4 - Reasoning

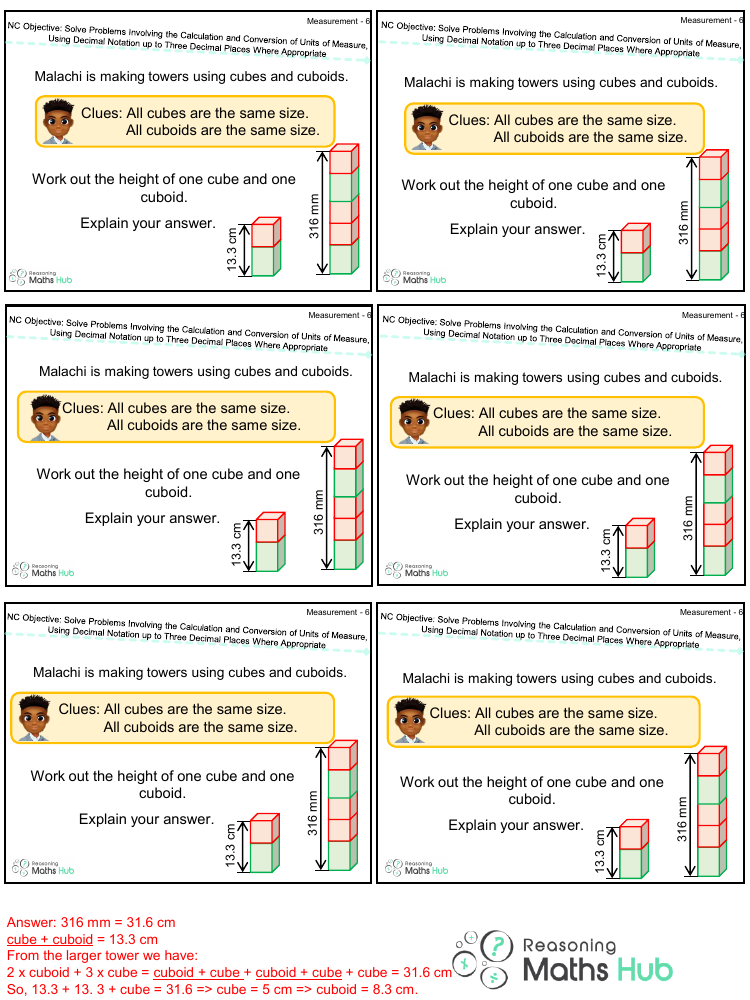
Malachi's task of constructing towers from cubes and cuboids presents an interesting problem involving the calculation and conversion of units of measure. Given that all cubes and cuboids are uniform in size, the challenge is to determine the individual heights of one cube and one cuboid. The information provided indicates that the combined height of a cube and a cuboid is 13.3 cm, and the total height of a larger tower composed of these shapes is 31.6 cm, which is equivalent to 316 mm after conversion.

To solve this, we analyze the larger tower's structure, which is made up of two cuboids and three cubes. The total height of this arrangement is given as 31.6 cm. Using the provided clue that a combination of one cube and one cuboid measures 13.3 cm, we can deduce that two such combinations (2 cuboids + 2 cubes) would measure 26.6 cm. Subtracting this from the total height of the tower (31.6 cm) leaves us with the height of one cube, which is 5 cm. Knowing the height of the cube allows us to calculate the height of the cuboid by subtracting the cube's height from the combined height of one cube and one cuboid (13.3 cm - 5 cm), resulting in the height of the cuboid being 8.3 cm. This logical approach to the problem demonstrates the importance of understanding the relationships between shapes and their dimensions when calculating and converting units of measure.