Round numbers to required accuracy

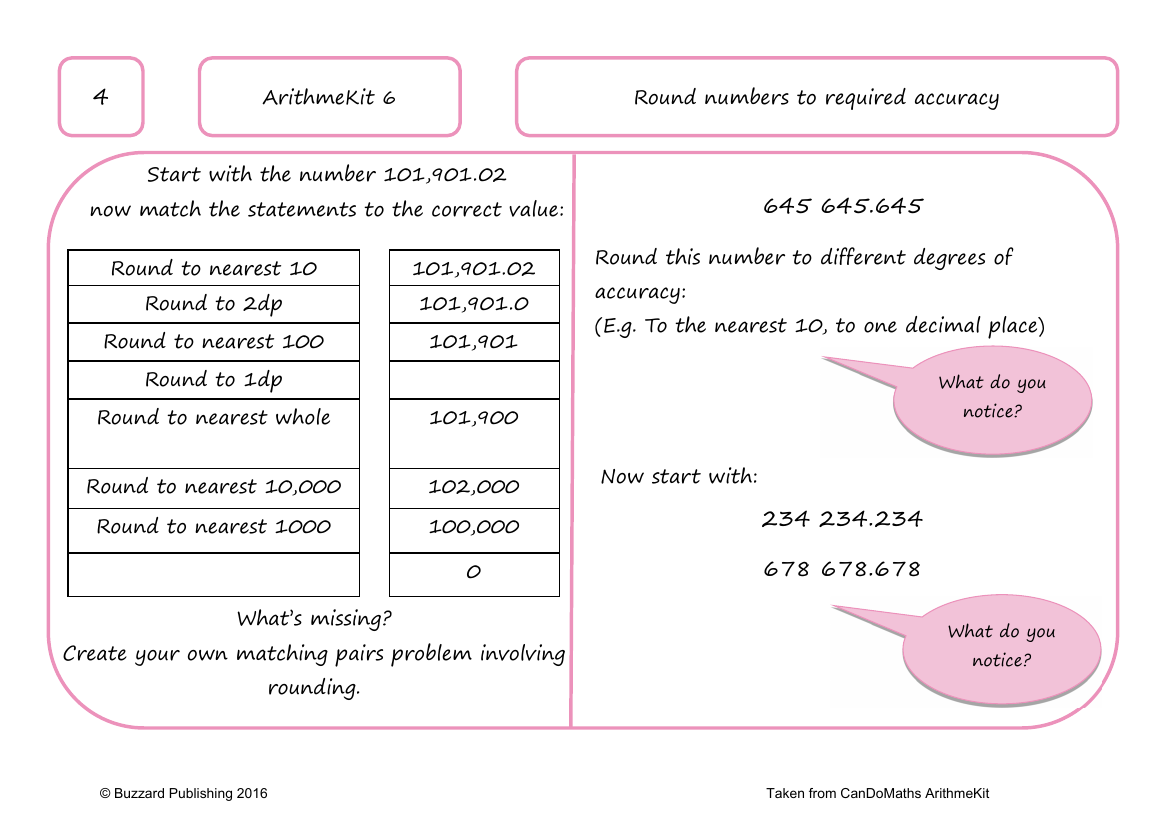
The ArithmeKit from Buzzard Publishing provides a comprehensive set of exercises for students to practise rounding numbers to various degrees of accuracy. For instance, when presented with the number 101,901.02, students are asked to round it to the nearest 10, resulting in 101,901.0, and to two decimal places (2dp), yielding the same outcome. Rounding to the nearest 100 gives 101,900, while rounding to the nearest 1,000 and 10,000 results in 102,000 and 100,000, respectively. These exercises encourage students to observe patterns in rounding and to understand the impact of different rounding intervals on the final number.

Additional tasks require students to round a variety of numbers to specified accuracies, such as rounding 43,867 to the nearest 10, which becomes 43,870, or rounding 656,798 to the nearest 10,000, resulting in 660,000. The activities cover a range of rounding scenarios, including to the nearest whole number, decimal places, and significant figures. For example, 67.987 rounded to one decimal place (1dp) is 68.0, and 0.9785 rounded to three decimal places (3dp) is 0.979. These exercises not only help students to master the concept of rounding but also to apply it in different contexts, enhancing their numerical fluency and problem-solving skills.