Multiplication and Division (1) - Multiply by 10 - Planning

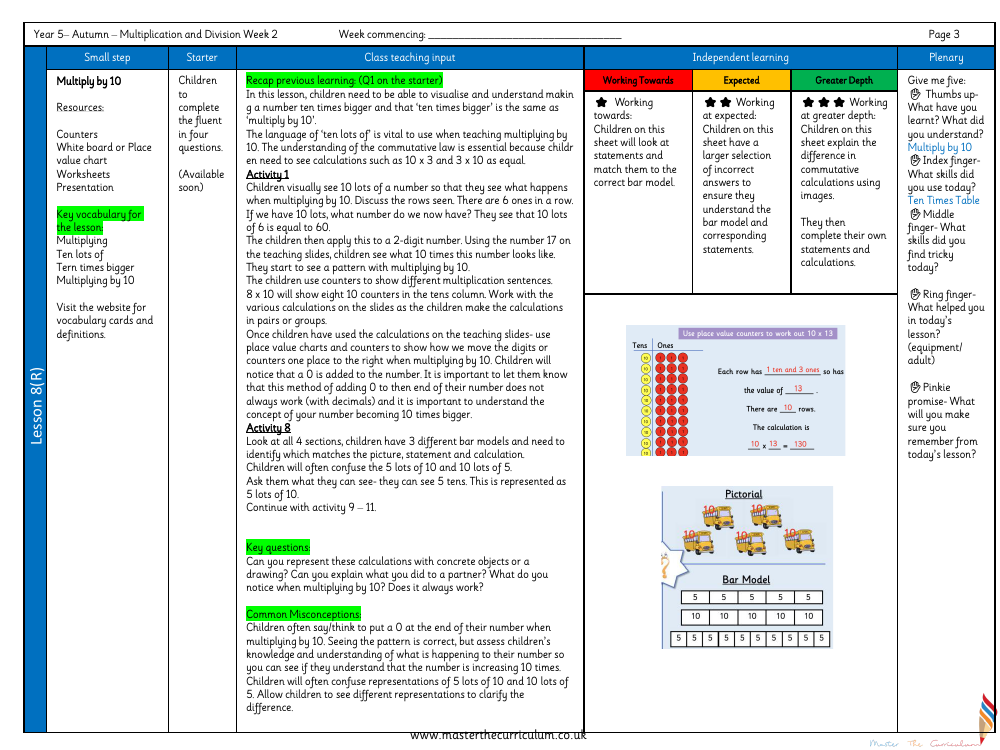
The lesson plan for Year 5 students on the topic of 'Multiply by 10' is designed to help them visualise and understand the concept of making a number ten times larger. The key vocabulary such as 'Multiplying', 'Ten lots of', and 'Ten times bigger' is introduced, and resources like counters, whiteboards or place value charts, worksheets, and a presentation are employed to facilitate learning. The lesson begins with a recap of previous learning where the equivalence of 'ten times bigger' and 'multiply by 10' is emphasised, along with the importance of using the language 'ten lots of'. The commutative law is also highlighted to help children understand that calculations like 10 x 3 and 3 x 10 yield the same result. During the first activity, children are shown what happens when a number is multiplied by 10, using visual aids and discussing the patterns observed with two-digit numbers. They use counters to create multiplication sentences and explore the concept further with place value charts, learning that digits move one place to the right when multiplied by 10, and a zero is added to the number—though they are cautioned this method does not always apply, such as with decimals.

Further activities involve using bar models to match pictures, statements, and calculations, addressing common misconceptions such as the tendency to simply add a zero to the end of a number when multiplying by 10. The lesson plan encourages questioning to assess understanding and to clarify the differences between expressions like '5 lots of 10' and '10 lots of 5'. The plenary session, 'Give me five', prompts students to reflect on what they have learned, the skills they used, and any difficulties they encountered. It also asks what helped them during the lesson and what they will remember moving forward. Differentiated tasks cater to varying levels of understanding, from 'Working towards' to 'Working at greater depth', where children match statements to bar models, ensure they comprehend the models and corresponding statements, and explain commutative calculations using images, ultimately leading to creating their own statements and calculations.