Compare and Order Numbers to at least 1,000,000 - Reasoning

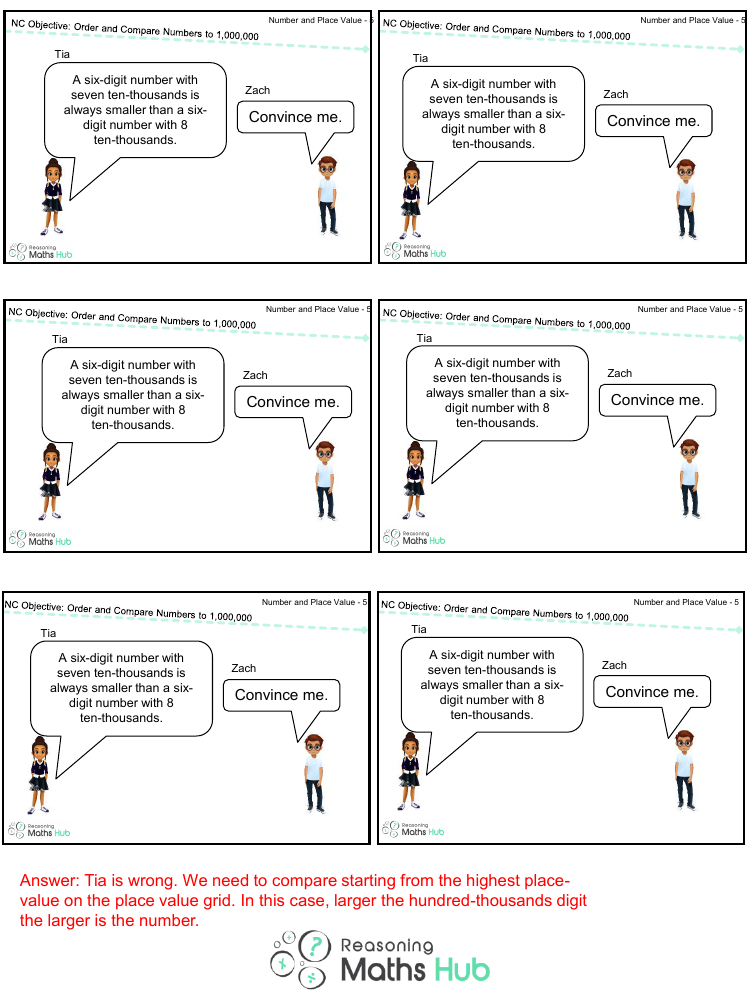
In a discussion about comparing and ordering numbers up to at least 1,000,000, the key concept is understanding the significance of place value. The dialogue presents a common misconception where Tia is corrected on her approach to comparing numbers. The correct method to determine which number is larger involves starting from the highest place value, which is the hundred-thousands place in a six-digit number. Therefore, a number with a digit of 8 in the ten-thousands place is always larger than one with a digit of 7 in the same place, irrespective of the other digits in the number.

Zach challenges Tia to convince him by reinforcing the rule of place value hierarchy. He asserts that when comparing six-digit numbers, the number with the larger ten-thousands digit is greater. This is because the ten-thousands place is the second-highest value position in a six-digit number, and thus has a greater impact on the overall value of the number than the places to its right. The repetition of the statement across the dialogue emphasizes the importance of this fundamental principle in number comparison and place value understanding.