Count in multiples of 6, 7, 9, 25 and 1000 - Reasoning

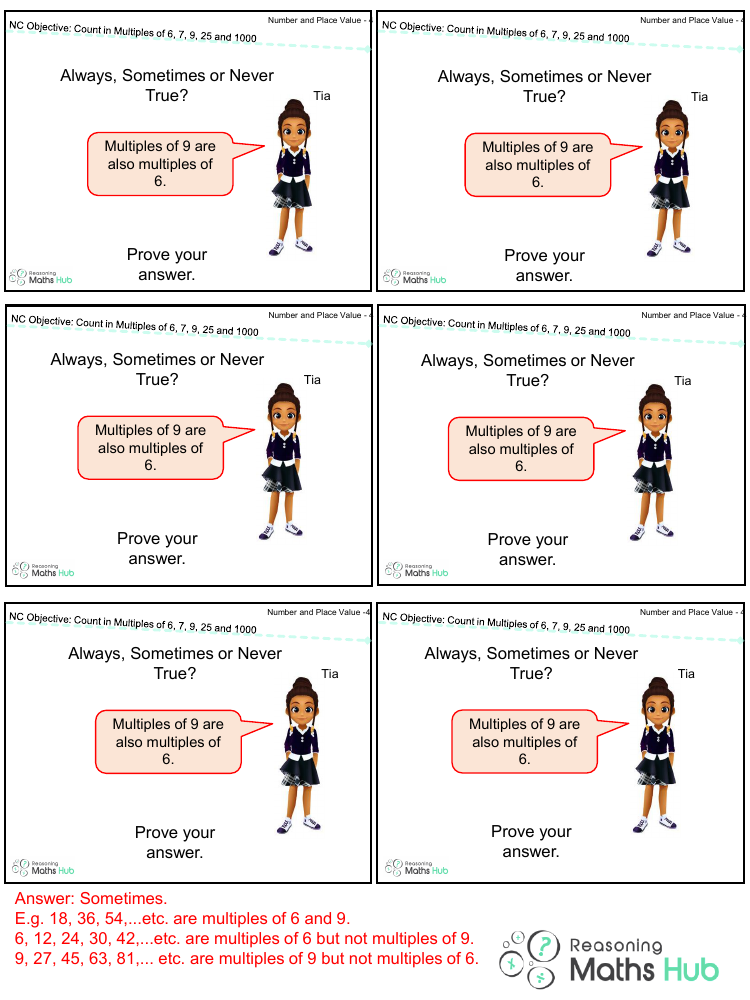
In the context of reasoning within the Number and Place Value unit, students are challenged to consider the relationship between multiples of different numbers, specifically 6 and 9. The question posed asks whether multiples of 9 are also multiples of 6, with the possible responses being 'Always', 'Sometimes', or 'Never'. The correct answer to this is 'Sometimes'. For instance, numbers like 18, 36, and 54 are indeed multiples of both 6 and 9. However, this is not always the case as shown by the sequence 6, 12, 24, 30, 42, which includes numbers that are multiples of 6 but not of 9. Conversely, there are numbers such as 9, 27, 45, 63, and 81, which are multiples of 9 but not of 6. Therefore, it is not universally true that all multiples of 9 are also multiples of 6.

To prove this, one can examine the factors of both numbers. Since 9 is not a multiple of 6, not all multiples of 9 will be multiples of 6. For a number to be a multiple of 6, it must be divisible by both 2 and 3. On the other hand, a multiple of 9 must be divisible by 9, which implies it must also be divisible by 3. Therefore, while all multiples of 9 will be divisible by 3, they will not necessarily be divisible by 2, and thus not all will be multiples of 6. This logical exploration confirms that the statement is only 'Sometimes' true, demonstrating the importance of critical thinking in understanding number relationships and place value.