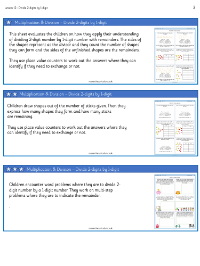
Multiplication and division - Divide two digits by one digit remainders - Presentation

The engaging lesson plan focuses on teaching Year 6 students how to divide two-digit numbers by one-digit numbers and understand the concept of remainders. The lesson begins with a discussion about what remainders are and why they occur in certain calculations. Students explore this concept through practical examples, such as determining the number of squares that can be made with a set number of lollipop sticks. For instance, the question posed is how to divide 13 lollipop sticks by 4, prompting students to identify the number of complete groups of 4 that can be formed and the number of sticks that would remain. This method is then applied to other scenarios, such as finding out how many triangles can be made with 38 lollipop sticks, where the calculation would be 38 ÷ 3, resulting in 12 complete groups with a remainder of 2.

Further activities encourage the use of different strategies to solve division problems with remainders. One such strategy is repeated subtraction, as demonstrated by a character named Zach, who subtracts the divisor repeatedly from the dividend until no more complete groups can be formed. For example, Zach uses this method to solve 31 ÷ 4, resulting in 7 complete groups with a remainder of 3. The lesson also introduces place value counters as a visual aid to help students understand division and remainders, such as dividing 94 by 4, which results in 23 complete groups with a remainder of 2. The lesson plan includes reasoning exercises that challenge students to identify odd calculations out and solve practical problems, like dividing stickers into equal groups. The lesson concludes with independent work, where students apply their knowledge to solve division questions and discuss the efficiency of different methods when dealing with larger two-digit numbers.